Refraction index

Article to be checked

Check of this article is requested.

Introduction

Refractive Index is a value commonly used in optical science. It has no unit and gives an indication of a materials ability to refract light. To understand the concept of Refractive index it is important to understand the concepts of refraction.

What is Refraction?

Refraction occurs when light or another type of radiation changes medium. When the light waves strike the new medium the atoms of the medium absorb the energy and re-radiate it at the same frequency, however there is a phase lag which causes the speed and wavelength to change.

This means there is a change in the direction of the wave. In the case of visible light, the light beam will either:

• move away from the normal (when going from a more dense medium to a less dense medium)
• or towards the normal (when going from a less dense medium to a more dense medium)

• 

  Refraction Through a Glass Block. (Click on the Image to Enlarge)

Refractive Index

The Refractive Index is a ratio between the speed of an electromagnetic wave in a vacuum and the speed of the same wave in another medium.

It gives an indication as to how much refraction will occur in a certain material. The refractive index through a vacuum is, by definition, 1.

If we define the normal as being the perpendicular line to the surface of the interface, then a higher value of refractive index will mean that the ray bends more towards the normal when passing from the vacuum to the medium.

Most refractive index values using visible light waves are between 1 and 2 whilst refractive index values using infrared waves may be much higher.

This clearly demonstrates that refractive index is affected by wavelength; as wavelength increases, the refractive index increases as well, as shown in the graph below representing the refractive index of water vs. wavelength of the wave:

• 

  Refractive Index of Water Vs. Wavelength of the Wave. (Click on the Image to Enlarge)

• 

  Spectrum Vs. Wavelength. (Click on the Image to Enlarge)

As we can observe in this graph, for short wavelength (ultraviolet), n is smaller than for long wavelengths (near Infrared spectrum). This means that the shorter wavelengths are refracted less than the longer wavelengths. For reference, the graph to the right shows the different spectrum vs. wavelength.

This dependency on wavelength can be used to explain the splitting of visible light when passing through a prism (this is called Dispersion) or the fact that the focal point of a lens changes with wavelength (this is called chromatic aberration).

Calculating Refractive Index

We know that in any given medium:

λ'×f=v

Where:

λ' is the wavelength in the medium

f is the frequency

v is the velocity

When applied to light passing through a vacuum the equation gives:

λ×f=c

Where:

λ is the wavelength in vacuum

f is the frequency

c is velocity of light through a vacuum (299,792,458m/s)

We know that the frequency does not change when a wave passes from one medium to another so we can combine these two equations to give the following:

λ/λ'=c/v

Or:

λ'=λ/(c⁄v)=λ/n

Where n is the ‘Refractive Index’, as defined above.

Snell’s Law

Snell’s Law states that:

n₁/n₂ =v₁/v₂ =sinθ₁/sinθ₂

Where:

1 represents the first medium

2 represents the second medium

n is the Refractive Index

v is the velocity

θ₁ = i = the angle between the normal and the incident ray

θ₂ = r = the angle between the normal and the refracted ray

• 

  Experiment to demonstrate Snell's Law. (Click on the Image to Enlarge)

This allows us to calculate the refractive index using the formula:

n=sini/sinr

Where the incident ray is in a vacuum medium

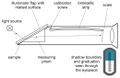
Measuring the index of refraction: Refractometers

A refractometer can be used to measure the refractive index of solids and liquids. It uses an LED light that shines through a prism onto the medium. Some light is refracted and some light is reflected back to the refractometer where the detectors pick this up. This allows the refractometer to calculate the critical angle (the angle at which light is no longer refracted, but is reflected instead) and hence deduce the refractive index.

• 

  Principle of a hand held refractometer, also called critical angle refractometer. (Click on the Image to Enlarge)

Principle of a hand held refractometer, also called critical angle refractometer

A substance can have different refractive indexes depending on concentration and temperature so both of these factors must be monitored when readings are being taken.

Clinical Applications

Diabetes

Refractometers can be used to determine the concentration of substances by using a standard known substance as a comparative. This can be used to measure the concentration of Glucose in the blood, which is useful when diagnosing and monitoring diabetes. Hand held refractometers exist for this use.

Ophthalmic

The difference in refractive index between the Cornea and the Lens is what enables the eye to concentrate light rays onto the retina. The Lens is also able to shift the fluid inside which can cause areas of increased thickness in either the optical axis (when looking at something close to you) or in the periphery (when looking at something farther away). This is accomplished by the Ciliary Muscles of the eye.

• 

  Adaptions of the eye for visualizing far and near objects. (Click on the Image to Enlarge)

One cause of Myopia is a higher index of refraction in the eye which causes the light rays to converge before the retina, hence the patient can only see objects that are nearby.

One cause of Hypermetropia there is a lower index of refraction in the eye which causes the light rays to converge past the retina, hence the patient can only see objects that are far away.

Refractometers can be used to diagnose Myopia and Hypermetropia by measuring the refractive index of the eye.

• 

  Using Refractometer to Diagnose Hypermetropia and Myopia. (Click on the Image to Enlarge)